Doppler effect: Difference between revisions
imported>John R. Brews (binary star) |
imported>John R. Brews (headers, sources) |
||
| Line 7: | Line 7: | ||
</ref> is the change in [[frequency]] of a [[wave]] for an observer moving relative to the source of the wave. If the observer moves oppositely to the direction of wave motion, the frequency appears higher, and if the observer moves in the same direction as the waves, the frequency appears lower. | </ref> is the change in [[frequency]] of a [[wave]] for an observer moving relative to the source of the wave. If the observer moves oppositely to the direction of wave motion, the frequency appears higher, and if the observer moves in the same direction as the waves, the frequency appears lower. | ||
==Analysis== | |||
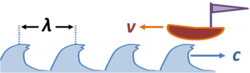
The increase in frequency when moving against the waves can be explained using the figure. Waves blowing ashore with velocity ''c'' are spaced a distance ''λ'' apart. Therefore, the time between crests for an observer at a fixed location is {{nowrap|''λ / c'',}} and the frequency with which a crest appears at a fixed location is ''f<sub>w</sub>'' : | The increase in frequency when moving against the waves can be explained using the figure. Waves blowing ashore with velocity ''c'' are spaced a distance ''λ'' apart. Therefore, the time between crests for an observer at a fixed location is {{nowrap|''λ / c'',}} and the frequency with which a crest appears at a fixed location is ''f<sub>w</sub>'' : | ||
| Line 25: | Line 26: | ||
Of course, if the boat runs inshore with the wave, the opposite happens: it takes the boat longer between crests, and the frequency with which the boat bumps over a crest is lower than the actual frequency of the waves. | Of course, if the boat runs inshore with the wave, the opposite happens: it takes the boat longer between crests, and the frequency with which the boat bumps over a crest is lower than the actual frequency of the waves. | ||
The same effect appears when the ear and a police siren move toward each other: the sound of the siren to the ear is pitched higher than the actual pitch of the siren, and when the police pass, so the ear and siren are separating, and the waves from the siren are traveling in the same direction as the ear, the pitch to the ear drops to become lower than the pitch of the siren. | ==Other examples== | ||
The same effect appears when the ear and a police siren move toward each other: the sound of the siren to the ear is pitched higher than the actual pitch of the siren, and when the police pass, so the ear and siren are separating, and the waves from the siren are traveling in the same direction as the ear, the pitch to the ear drops to become lower than the pitch of the siren.<ref name=pitch> | |||
As for binary stars, the case considered by Doppler, as the stars orbit each other, unless they are in a plane perpendicular to the line of sight, they are alternately moving toward and away from the observing astronomer, so the frequency of the light from each star (its color) alternates as well. | For a discussion see {{cite book |title=In Quest of the Solar System |author=Theo Koupelis |url=http://books.google.com/books?id=WRiW8-U9EssC&pg=PA115 |pages=pp. 115 ''ff'' |chapter=§4–7: The Doppler effect |isbn=0763766291 |year=2010 |publisher=Jones & Bartlett Learning}} | ||
</ref> | |||
As for binary stars, the case considered by Doppler, as the stars orbit each other, unless they are in a plane perpendicular to the line of sight, they are alternately moving toward and away from the observing astronomer, so the frequency of the light from each star (its color) alternates as well.<ref name=binary> | |||
For a discussion see {{cite book |title=Discovering the Universe: From the Stars to the Planets |author=Neil F. Comins, William J. Kaufmann |url=http://books.google.com/books?id=J1d9HJHlISkC&pg=PA174 |pages=pp. 174 ''ff'' |chapter=§6–12: The orbital motion of binary stars affects the wavelengths of their spectral lines |isbn=1429230428 |year=2008 |publisher=Macmillan}} | |||
</ref> | |||
==Notes== | ==Notes== | ||
<references/> | <references/> | ||
Revision as of 07:49, 13 April 2011
The Doppler effect (or Doppler shift, or Doppler's principle), named after Christian Doppler who proposed it in 1842,[1] is the change in frequency of a wave for an observer moving relative to the source of the wave. If the observer moves oppositely to the direction of wave motion, the frequency appears higher, and if the observer moves in the same direction as the waves, the frequency appears lower.
Analysis
The increase in frequency when moving against the waves can be explained using the figure. Waves blowing ashore with velocity c are spaced a distance λ apart. Therefore, the time between crests for an observer at a fixed location is λ / c, and the frequency with which a crest appears at a fixed location is fw :
The boat going to sea is running at velocity v in the opposite direction to the waves. Consequently the boat moves relative to the crests at a speed v+c. That means a crest is met at intervals of time τ :
In other words, the frequency with which the boat bumps over a crest fb is:
so evidently fb is a higher frequency than the frequency fw of the waves themselves. This increase in frequency is the Doppler effect, and it is often expressed as the shift in frequency fd, the Doppler shift, namely:
Of course, if the boat runs inshore with the wave, the opposite happens: it takes the boat longer between crests, and the frequency with which the boat bumps over a crest is lower than the actual frequency of the waves.
Other examples
The same effect appears when the ear and a police siren move toward each other: the sound of the siren to the ear is pitched higher than the actual pitch of the siren, and when the police pass, so the ear and siren are separating, and the waves from the siren are traveling in the same direction as the ear, the pitch to the ear drops to become lower than the pitch of the siren.[2]
As for binary stars, the case considered by Doppler, as the stars orbit each other, unless they are in a plane perpendicular to the line of sight, they are alternately moving toward and away from the observing astronomer, so the frequency of the light from each star (its color) alternates as well.[3]
Notes
- ↑ C Doppler (1843). "Über das farbige Licht der Doppelsterne und einiger anderer Gestirne des Himmels (On the colored light of the binary stars and some other stars of the heavens)". Abhandlungen der koniglich bohmischen Gesellschaft der Wissenschaften vol 2,: pp. 465-482.
- ↑ For a discussion see Theo Koupelis (2010). “§4–7: The Doppler effect”, In Quest of the Solar System. Jones & Bartlett Learning, pp. 115 ff. ISBN 0763766291.
- ↑ For a discussion see Neil F. Comins, William J. Kaufmann (2008). “§6–12: The orbital motion of binary stars affects the wavelengths of their spectral lines”, Discovering the Universe: From the Stars to the Planets. Macmillan, pp. 174 ff. ISBN 1429230428.