Legendre-Gauss Quadrature formula: Difference between revisions
imported>Dmitrii Kouznetsov (Did not finish yet.) |
imported>Dmitrii Kouznetsov m (→Nodes and weights: mistypes) |
||
| Line 7: | Line 7: | ||
Legendre-Gauss quadratude formula is special case of [[Gaussian quadratures]] of more general kind, which allow efficient approximation of a function with known asumptiotic behavior at the edges of the interval of integration. | Legendre-Gauss quadratude formula is special case of [[Gaussian quadratures]] of more general kind, which allow efficient approximation of a function with known asumptiotic behavior at the edges of the interval of integration. | ||
==Nodes and weights== | ==Nodes and weights== | ||
Nodes <math>x_i</math> in equation (1) are zeros of the [[ | Nodes <math>x_i</math> in equation (1) are zeros of the [[Polynomial of Legendre]] <math>P_N</math>: | ||
: (2) <math> P_N(x_i)=0</math> | : (2) <math> P_N(x_i)=0</math> | ||
: (3) <math> -1<x_1<x_2< ... <x_N <1</math> | : (3) <math> -1<x_1<x_2< ... <x_N <1</math> | ||
| Line 31: | Line 31: | ||
|publisher=[[Do not forget to type data of the ref. here!]] | |publisher=[[Do not forget to type data of the ref. here!]] | ||
}}</ref> | }}</ref> | ||
==Precision of the approximation== | ==Precision of the approximation== | ||
Revision as of 08:40, 27 May 2008
Legendre-Gauss Quadratude formiula is the approximation of the integral
- (1)
with special choice of nodes and weights , characterised in that, if the finction is polynomial of order smallet than , then the exact equality takes place in equation (1).
Legendre-Gauss quadratude formula is special case of Gaussian quadratures of more general kind, which allow efficient approximation of a function with known asumptiotic behavior at the edges of the interval of integration.
Nodes and weights
Nodes in equation (1) are zeros of the Polynomial of Legendre :
- (2)
- (3)
Weight in equaiton (1) can be expressed with
- (4)
There is no straightforward espression for the nodes ; they can be approximated with many decimal digits through only few iterations, solving numerically equation (2) with initial approach
- (5)
These formulas are described in the books [1] [2]
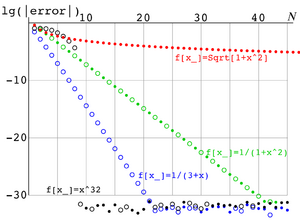
Precision of the approximation
Example
Extension to other interval
is straightforward. Should I copypast the obvious formulas here?
References
- ↑ (1980) Handbook on special functions.
- ↑ Numerical Resipes in C. Do not forget to type data of the ref. here!.