Polyhedron: Difference between revisions
imported>Anthony Argyriou (create) |
imported>Anthony Argyriou (add images and data to table) |
||
| Line 8: | Line 8: | ||
{| class=wikitable style="font-size: normal; font-style: normal; align: left; width: 100%" | {| class=wikitable style="font-size: normal; font-style: normal; align: left; width: 100%" | ||
|- align="left" | |- align="left" | ||
!number of faces!!name!!type of face!!properties | !number<br />of<br />faces!!name!!type of face!!properties!!image | ||
|- | |- | ||
|4 | |4 | ||
|[[regular tetrahedron]] (or regular triangular pyramid) | |[[regular tetrahedron]]<br />(or regular triangular pyramid) | ||
|equilateral [[triangle]] | |equilateral [[triangle]] | ||
| | |4 vertices, 6 edges, self-dual | ||
|[[Image:Tetrahedron.png]] | |||
|- | |- | ||
|6 | |6 | ||
|[[cube]] | |[[cube]] | ||
|[[square]] | |[[square]] | ||
| | |8 vertices, 12 edges, dual to octahedron | ||
|[[Image:Cube.png]] | |||
|- | |- | ||
|8 | |8 | ||
|[[regular octahedron]] | |[[regular octahedron]] | ||
|equilateral [[triangle]] | |equilateral [[triangle]] | ||
| | |6 vertices, 12 edges, dual to cube | ||
|[[Image:Octahedron.png]] | |||
|- | |- | ||
|12 | |12 | ||
|[[regular dodecahedron]] | |[[regular dodecahedron]] | ||
|regular [[pentagon]] | |regular [[pentagon]] | ||
| | |20 vertices, 30 edges, dual to icosahedron | ||
|[[Image:Dodecahedron.png]] | |||
|- | |- | ||
|20 | |20 | ||
|[[regular icosahedron]] | |[[regular icosahedron]] | ||
|equilateral [[triangle]] | |equilateral [[triangle]] | ||
| | |12 vertices, 30 edges, dual to dodecahedron | ||
|[[Image:Icosahedron.png]] | |||
|- | |- | ||
|} | |} | ||
Revision as of 22:08, 14 October 2008
A polyhedron is a three-dimensional geometric closed figure bounded by a connected set of polygons. A polyhedron, in Euclidian geometry, must have at least four faces. A polyhedron of four sides is called a tetrahedron, six sides a hexahedron, eight sides an octahedron, ten sides a decahedron. Figures with more sides are typically named with the Greek name for the number of sides, followed by "-hedron".
The polygons bounding a polyhedron are known as faces; the line segments bounding the polygons are known as edges, and the points where the faces meet are vertices (singular vertex).
A convex polyhedron bounded by faces which are all the same-sized regular polygon is known as a Platonic solid. There are only five Platonic solids, shown in the table below:
| number of faces |
name | type of face | properties | image |
|---|---|---|---|---|
| 4 | regular tetrahedron (or regular triangular pyramid) |
equilateral triangle | 4 vertices, 6 edges, self-dual | 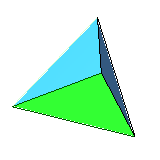
|
| 6 | cube | square | 8 vertices, 12 edges, dual to octahedron | 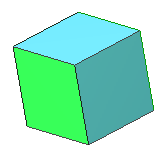
|
| 8 | regular octahedron | equilateral triangle | 6 vertices, 12 edges, dual to cube | 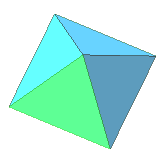
|
| 12 | regular dodecahedron | regular pentagon | 20 vertices, 30 edges, dual to icosahedron | 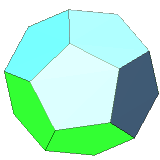
|
| 20 | regular icosahedron | equilateral triangle | 12 vertices, 30 edges, dual to dodecahedron | 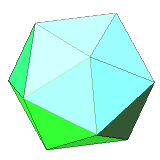
|