Doppler effect

The Doppler effect (or Doppler shift, or Doppler's principle), named after Christian Doppler who proposed it in 1842, is the change in frequency of a wave for an observer moving relative to the source of the wave. If the observer and source of wave motion approach each other, the frequency appears higher, and if the observer and the source separate, the frequency appears lower.

Analysis

(PD) Image: John R. Brews
Boat traveling against waves experiences Doppler effect.

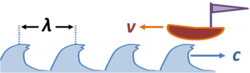
The increase in frequency when moving toward a source can be explained using the figure. Suppose that waves blowing ashore with velocity c are spaced a distance λ apart. In that case, the time between crests for an observer at a fixed location is λ / c, and the frequency with which a crest appears at a fixed location is f_w :

$f_{w}={\frac {1}{\lambda /c}}={\frac {c}{\lambda }}\ .$

The boat going to sea is running at velocity v in the opposite direction to the waves, and toward the source of the wind. Consequently the boat moves relative to the crests at a speed v+c. That means a crest is met at intervals of time τ :

$\tau ={\frac {\lambda }{(v+c)}}\ .$

In other words, the frequency with which the boat bumps over a crest f_b is:

$f_{b}={\frac {1}{\tau }}={\frac {1}{\lambda /(v+c)}}=f_{w}{\frac {v+c}{c}}=f_{w}\left(1+{\frac {v}{c}}\right)\ .$

so evidently f_b is a higher frequency than the frequency f_w of the waves themselves. This increase in frequency is the Doppler effect, and it is often expressed as the shift in frequency f_d, the Doppler shift, namely:

$f_{d}=f_{b}-f_{w}=f_{w}{\frac {v}{c}}\ .$

Of course, if the boat runs inshore with the wave, away from the source of the wind, the opposite happens: it takes the boat longer between crests, and the frequency with which the boat bumps over a crest is lower than the actual frequency of the waves. In particular, if the boat moves at the same speed as the waves (rides the waves into shore) the frequency of bumping over crests drops to zero.

(PD) Image: John R. Brews
Pulse separation for a stationary source (top) and a moving source (bottom)

One might think it immaterial whether the source approached the observer, or the other way around. However, it is not that simple.

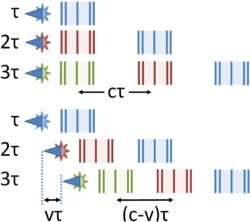
The figure at the right shows a source emitting pulses every τ seconds that propagate at a speed c. The distance between pulses when the source is stationary is λ_s = cτ. However, when the source moves with a speed v, it translates a distance vτ between pulses, moving each pulse closer to its predecessor by this distance, and resulting in a spacing between pulses of λ_m = (c−v)τ. Consequently the wave with a moving source has a frequency f_m related to the frequency when the source is stationary f_s as:

$f_{m}=f_{s}{\frac {c}{c-v}}=f_{s}{\frac {1}{1-v/c}}\ ,$

and a Doppler shift f_d of

$f_{d}=f_{s}{\frac {v/c}{1-v/c}}\ .$

If the source moves as fast as the pulses, so v=c, this frequency becomes infinite, and the interpretation is that the source cannot emit a pulse in the forward direction because it always is moving ahead of the pulse.

In the general case, the observed frequency f_o is related to the emission frequency of the source f_e as:

$f_{o}=f_{e}\left({\frac {1+v_{o}/c}{1-v_{s}/c}}\right)\ ,$

with c the speed of advance of the wave, v_o the speed of the observer moving against the wave direction, and v_s the speed of the source moving in the wave direction. For positive v’s, that is, with both the observer and the source moving toward each other, both the numerator (>1) and the denominator (<1) increase the observed frequency.

Absolute motion

It would appear that careful observation of the Doppler effect could distinguish between movement of the source and that of the observer. But if they are in uniform straight-line motion toward one another, should it be possible to distinguish which object was moving? The answer is "yes, we can tell the difference" in the case where a medium is involved like air or water, because the medium introduces a third reference frame, and the discussion above is made in the frame of the medium. However, in the case of light in a classical vacuum, according to special relativity one cannot detect the medium, so the Doppler shift cannot be given by the above formula. The idea resolving this paradox was pointed out by Einstein; time dilation: when the source or the observer moves, their internal clocks slow down, an effect noticeable as speeds approach the speed of light. In the case of light, the Doppler effect cannot distinguish whether the source or the observer is moving, only that they are approaching each other or separating from each other. Consequently light in classical vacuum is a special case.

An interesting corollary of special relativity is that there is a transverse Doppler effect for light emitted in vacuum, that is, a Doppler effect is present even for an observation perpendicular to the motions of source or observer, sometimes called the second-order Doppler effect because it is of second order, varying as (v/c)².

Other examples

The same effect appears when the ear and a police siren move toward each other: the sound of the siren to the ear is pitched higher than the actual pitch of the siren, and when the police pass, so the ear and siren are separating, and the waves from the siren are traveling in the same direction as the ear, the pitch to the ear drops to become lower than the pitch of the siren.

As for binary stars, the case considered by Doppler, as the stars orbit each other, they are alternately moving toward and away from the observing astronomer, so the frequency of the light from each star (its color) alternates as well. A Doppler effect is seen for stars even when they are in a plane perpendicular to the line of sight, because of the transverse Doppler effect predicted by special relativity. In this example, the light always propagates in the same direction, and only the separation of the observer and source is changing.