Iteration of function: Difference between revisions
imported>Dmitrii Kouznetsov (copypast from TORI, convert $ to <math> or </math> Problem with "atop") |
imported>Roger A. Lohmann m (add metadata & subpages) |
||
| (14 intermediate revisions by 3 users not shown) | |||
| Line 1: | Line 1: | ||
{{subpages}} | |||
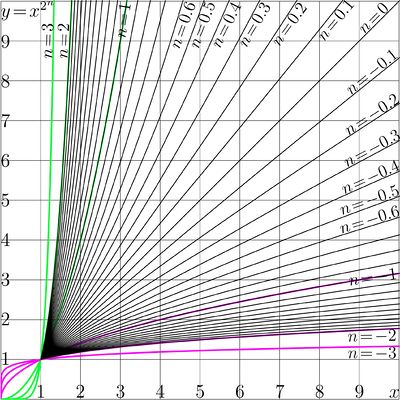
[[File:PowIteT.jpg|400px|thumb|Fig.1. Iterates of <math>T(z)=z^2~</math>: <math>~y\!=\!T^n(x)\!=\!x^{2^n}~</math> for various <math>n</math>]] | [[File:PowIteT.jpg|400px|thumb|Fig.1. Iterates of <math>T(z)=z^2~</math>: <math>~y\!=\!T^n(x)\!=\!x^{2^n}~</math> for various <math>n</math>]] | ||
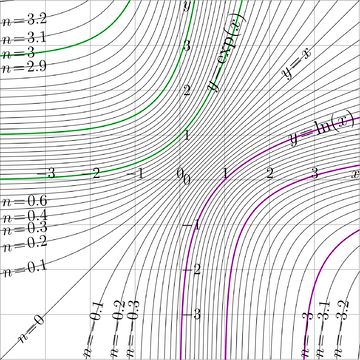
[[File:ExpIte4T.jpg|360px|thumb|Fig.2. Iterates of <math>T(z)=\exp(z)~</math>: <math>~y\!=\!T^n(x)\!=\!\exp^n(x)~</math> for various <math>n</math>]] | [[File:ExpIte4T.jpg|360px|thumb|Fig.2. Iterates of <math>T(z)=\exp(z)~</math>: <math>~y\!=\!T^n(x)\!=\!\exp^n(x)~</math> for various <math>n</math>]] | ||
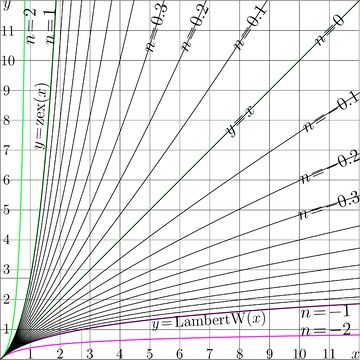
[[File:ZexIteT.jpg|360px|thumb|Fig.3. Iterates of <math>T(z)=z\exp(z)~</math>: <math>~y\!=\!T^n(x)\!=\!\mathrm{zex}^n(x)~</math> for various <math>n</math>]] | [[File:ZexIteT.jpg|360px|thumb|Fig.3. Iterates of <math>T(z)=z\exp(z)~</math>: <math>~y\!=\!T^n(x)\!=\!\mathrm{zex}^n(x)~</math> for various <math>n</math>]] | ||
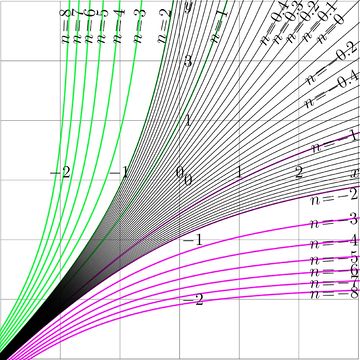
[[File:TraItT.jpg|360px|thumb|Fig.4. Iterates of <math>T(z)=z+\exp(z)~</math>: <math>~y\!=\!T^n(x)\!=\!\mathrm{tra}^n(x)~</math> for various <math>n</math>]] | [[File:TraItT.jpg|360px|thumb|Fig.4. Iterates of <math>T(z)=z+\exp(z)~</math>: <math>~y\!=\!T^n(x)\!=\!\mathrm{tra}^n(x)~</math> for various <math>n</math>]] | ||
The '''iterate of a function''' , or '''iterate''' ([[итерация]]), is a function, expressed as repetition of another (iterated) function; the latter may be called the [[iterand]]. | |||
Any function by itself is considered as its first iterate. | Any function by itself is considered as its first iterate. | ||
The zeroth iterate of a function is | The zeroth iterate of a function is considered to be the [[identity function]]. | ||
The minus first iteration of a function is its [[inverse function]] | The minus first iteration of a function is its [[inverse function]] | ||
In general, iteration of a function <math>T</math> is denoted with superscript; <math>T^n</math> means the <math>n</math>th iteration of function <math>T</math>, i.e., | |||
(1) <math>~ ~ ~ \displaystyle T^n(z) =T\!\Big(T\!\big( ... T(z)... \big)\Big)</math> | |||
<math> | where the function <math>T</math> in the right hand side of equation is repeated <math>n</math> times. (Note, however, that this abstract notation is not always used consistently for actual functions: for example, cos<sup>2</sup>θ means (cos θ)<sup>2</sup>, not cos cos θ, by convention.) | ||
This may be expressed with equations | This may be expressed with the equations | ||
<math>~T^0(z)\!=\!z~</math>, <math>~ T^n(z)=T\Big( T^{n-1}(z) \Big)~</math> for <math>n\! > \!0</math> | (2) <math>~ ~ ~ T^0(z)\!=\!z~</math>, <math>~ T^n(z)=T\Big( T^{n-1}(z) \Big)~</math> for <math>n\! > \!0</math> | ||
Term [[iteration]] may have also another meaning, indicating repetition of some process (even if such a repetition does not define a new function). | Term [[iteration]] may have also another meaning, indicating repetition of some process (even if such a repetition does not define a new function). | ||
| Line 37: | Line 39: | ||
With [[Nest]], the iteration of function <math>f</math> can be written as follows: | With [[Nest]], the iteration of function <math>f</math> can be written as follows: | ||
<math> f^n(z)=\mathrm{Nest}[f,z,n]</math> | (3) <math>~ ~ ~ f^n(z)=\mathrm{Nest}[f,z,n]</math> | ||
In [[Mathematica]], all arguments of all functions should be written in squared parenthesis. Up to year 2012, the [[Nest]] is implemented only for the cases, when number <math>n</math> of iterations can be simplified to a [[natural number]], expressed with integer constant. Attempts to call [[Nest]] to evaluate a non–integer iterate of a function are interpreted as error. | In [[Mathematica]], all arguments of all functions should be written in squared parenthesis. Up to year 2012, the [[Nest]] is implemented only for the cases, when number <math>n</math> of iterations can be simplified to a [[natural number]], expressed with integer constant. Attempts to call [[Nest]] to evaluate a non–integer iterate of a function are interpreted as error. | ||
| Line 45: | Line 47: | ||
Let <math>T(z)=a+b z</math>. Then | Let <math>T(z)=a+b z</math>. Then | ||
<math>\displaystyle T^n(z)=a \frac{1\!-\!b^n}{1\!-\!b} + b^n z</math> | (4)<math>~ ~ ~\displaystyle T^n(z)=a \frac{1\!-\!b^n}{1\!-\!b} + b^n z</math> | ||
This expression allows the straightforward interpretation for non–integer <math>n</math>. | This expression allows the straightforward interpretation for non–integer <math>n</math>. | ||
The case of [[power function]] is also simple. Let <math>T(z)=z^a</math>, where <math>a\!>\!1</math>. | The case of [[power function]] is also simple. Let <math>T(z)=z^a</math>, where <math>a\!>\!1</math>. | ||
| Line 54: | Line 55: | ||
In this expression, <math>n</math> has no need to be integer. | In this expression, <math>n</math> has no need to be integer. | ||
For <math>a\!=\!2</math>, the iterations of quadratic function are shown in Figure 1. | For <math>a\!=\!2</math>, the iterations of quadratic function are shown in Figure 1. | ||
For more general case, the non–integer iterates of a function <math>T</math> can be defined through its [[superfunction]] <math>F</math> and the [[Abel function]] <math>G\!=\! F^{-1}</math>; the iterand <math>T</math> is treated as the [[transfer function]], and the [[superfunction]] <math>F</math> satisfies the transfer equation | For more general case, the non–integer iterates of a function <math>T</math> can be defined through its [[superfunction]] <math>F</math> and the [[Abel function]] <math>G\!=\! F^{-1}</math>; the iterand <math>T</math> is treated as the [[transfer function]], and the [[superfunction]] <math>F</math> satisfies the transfer equation | ||
<math>F(z\!+\!1)=T\!\Big( F(z) \Big)</math> | (5)<math>~ ~ ~ F(z\!+\!1)=T\!\Big( F(z) \Big)</math> | ||
Then, the <math>n</math>th iteration of the transfer function can be expressed as | Then, the <math>n</math>th iteration of the transfer function can be expressed as | ||
<math>T^n(z)=F\Big(n+G(z) \Big)</math> | (6)<math>~ ~ ~ T^n(z)=F\Big(n+G(z) \Big)</math> | ||
In this expression, parameter <math>n</math> also has no need to be integer. Once holomorphic functions <math>F</math> and <math>G</math> are established, the function can be iterated even complex number of times. | In this expression, parameter <math>n</math> also has no need to be integer. Once holomorphic functions <math>F</math> and <math>G</math> are established, the function can be iterated even complex number of times. | ||
For a given [[transfer function]] <math>T</math>, the establishment of the | For a given [[transfer function]] <math>T</math>, the establishment of the superfunction <math>F</math> and the Abel function <math>G</math> is not trivial, but solvable problem. It had been formulated in 1950 by [[Helmuth Knezer]] | ||
<ref>http://tori.ils.uec.ac.jp/PAPERS/Relle.pdf | <ref>http://tori.ils.uec.ac.jp/PAPERS/Relle.pdf | ||
H.Kneser. "Reelle analytische Loesungen der Gleichung f(f(x))=ex und verwandter Funktionalgleichungen". Journal fur die Reine und Angewandte Mathematik 187 (1950): 56-67. | H.Kneser. "Reelle analytische Loesungen der Gleichung f(f(x))=ex und verwandter Funktionalgleichungen". Journal fur die Reine und Angewandte Mathematik 187 (1950): 56-67. | ||
</ref> | </ref> | ||
for iterates of [[exponential]], the half-iterate of the exponential appears in the title of his article. In century 21, such a function had been calculated and plotted | for iterates of [[exponential]], the half-iterate of the exponential appears in the title of his article. In century 21, such a function had been calculated and plotted | ||
<ref> | <ref name="analuxp"> | ||
http://www.ams.org/mcom/2009-78-267/S0025-5718-09-02188-7/home.html | http://www.ams.org/mcom/2009-78-267/S0025-5718-09-02188-7/home.html | ||
Preprint: http://www.ils.uec.ac.jp/~dima/PAPERS/2009analuxpRepri.pdf | Preprint: http://www.ils.uec.ac.jp/~dima/PAPERS/2009analuxpRepri.pdf | ||
| Line 76: | Line 78: | ||
http://tori.ils.uec.ac.jp/PAPERS/2010vladie.pdf | http://tori.ils.uec.ac.jp/PAPERS/2010vladie.pdf | ||
D.Kouznetsov. Superexponential as special function. Vladikavkaz Mathematical Journal, 2010, v.12, issue 2, p.31-45. | D.Kouznetsov. Superexponential as special function. Vladikavkaz Mathematical Journal, 2010, v.12, issue 2, p.31-45. | ||
</ref>; the iterates of the exponential are shown in Figure 2. Then, the formalism had been extended to other functions | </ref>; the iterates of the exponential are shown in Figure 2. Then, the formalism had been extended to other functions, in particular, to the exponentials to other bases | ||
<ref> | <ref name="sqrt2"> | ||
http://www.ams.org/journals/mcom/2010-79-271/S0025-5718-10-02342-2/home.html | |||
http://tori.ils.uec.ac.jp/PAPERS/2010sqrt2.pdf | |||
http://tori.ils.uec.ac.jp/PAPERS/2010q2.pdf | |||
D.Kouznetsov, H.Trappmann. Portrait of the four regular super-exponentials to base sqrt(2). Mathematics of Computation, 2010, v.79, p.1727-1756. | |||
</ref><ref name="e1e"> | |||
http://www.ams.org/journals/mcom/0000-000-00/S0025-5718-2012-02590-7/S0025-5718-2012-02590-7.pdf | |||
http://tori.ils.uec.ac.jp/PAPERS/2012e1eMcom2590.pdf | |||
H.Trappmann, D.Kouznetsov. Computation of the Two Regular Super-Exponentials to base exp(1/e). (Mathematics of Computation, 2012 February 8.) ISSN 1088-6842(e) ISSN 0025-5718(p) | |||
</ref>, [[factorial]] <ref name="factorial"> | |||
http://www.springerlink.com/content/qt31671237421111/fulltext.pdf?page=1 | |||
http://tori.ils.uec.ac.jp/PAPERS/2010superfae.pdf | |||
D.Kouznetsov, H.Trappmann. Superfunctions and square root of factorial. Moscow University Physics Bulletin, 2010, v.65, No.1, p.6-12. (Russian version: p.8-14) | |||
</ref>and the [[logistic sequence]] | |||
<ref name="logistic"> http://tori.ils.uec.ac.jp/PAPERS/2010logistie.pdf | |||
http://www.springerlink.com/content/u712vtp4122544x4 | |||
D.Kouznetsov. Holomorphic extension of the logistic sequence. Moscow University Physics Bulletin, 2010, No.2, p.91-98. (Russian version: p.24-31) DOI 10.3103/S0027134910020049 | |||
</ref>. | |||
For the non–integer iterates, it should be assumed that <math>T</math> is [[holomorphic function]], and the superfunction <math>G</math> is also expected to be holomorphic. In order to provide uniqueness of the superfunction, the certain requirements on its behavior should be assumed. Usually, <math>F</math> approaches some [[fixed point]]s of function <math>T</math> at infinity (see the special articles [[Superfunction]] and [[Abel function]]). | |||
Usually, the physically–meaningful [[transfer function]]s have a real [[fixed point]] and the corresponding [[superfunction]] and the [[Abel function]] can be evaluated with the [[regular iteration]] | |||
<ref name="sqrt2"> | |||
http://www.ams.org/journals/mcom/2010-79-271/S0025-5718-10-02342-2/home.html | http://www.ams.org/journals/mcom/2010-79-271/S0025-5718-10-02342-2/home.html | ||
http://tori.ils.uec.ac.jp/PAPERS/2010sqrt2.pdf | http://tori.ils.uec.ac.jp/PAPERS/2010sqrt2.pdf | ||
http://tori.ils.uec.ac.jp/PAPERS/2010q2.pdf | http://tori.ils.uec.ac.jp/PAPERS/2010q2.pdf | ||
D.Kouznetsov, H.Trappmann. Portrait of the four regular super-exponentials to base sqrt(2). Mathematics of Computation, 2010, v.79, p.1727-1756. | D.Kouznetsov, H.Trappmann. Portrait of the four regular super-exponentials to base sqrt(2). Mathematics of Computation, 2010, v.79, p.1727-1756. | ||
</ref><ref> | </ref><ref name="e1e"> | ||
http://www.ams.org/journals/mcom/0000-000-00/S0025-5718-2012-02590-7/S0025-5718-2012-02590-7.pdf | http://www.ams.org/journals/mcom/0000-000-00/S0025-5718-2012-02590-7/S0025-5718-2012-02590-7.pdf | ||
http://tori.ils.uec.ac.jp/PAPERS/2012e1eMcom2590.pdf | http://tori.ils.uec.ac.jp/PAPERS/2012e1eMcom2590.pdf | ||
H.Trappmann, D.Kouznetsov. Computation of the Two Regular Super-Exponentials to base exp(1/e). (Mathematics of Computation, 2012 February 8.) ISSN 1088-6842(e) ISSN 0025-5718(p) | H.Trappmann, D.Kouznetsov. Computation of the Two Regular Super-Exponentials to base exp(1/e). (Mathematics of Computation, 2012 February 8.) ISSN 1088-6842(e) ISSN 0025-5718(p) | ||
</ref><ref name="factorial"> | </ref>, [[factorial]] <ref name="factorial"> | ||
http://www.springerlink.com/content/qt31671237421111/fulltext.pdf?page=1 | http://www.springerlink.com/content/qt31671237421111/fulltext.pdf?page=1 | ||
http://tori.ils.uec.ac.jp/PAPERS/2010superfae.pdf | http://tori.ils.uec.ac.jp/PAPERS/2010superfae.pdf | ||
D.Kouznetsov, H.Trappmann. Superfunctions and square root of factorial. Moscow University Physics Bulletin, 2010, v.65, No.1, p.6-12. (Russian version: p.8-14) | D.Kouznetsov, H.Trappmann. Superfunctions and square root of factorial. Moscow University Physics Bulletin, 2010, v.65, No.1, p.6-12. (Russian version: p.8-14) | ||
</ref>, | </ref>and the [[logistic sequence]] | ||
<ref name="logistic"> http://tori.ils.uec.ac.jp/PAPERS/2010logistie.pdf | |||
http://www.springerlink.com/content/u712vtp4122544x4 | |||
D.Kouznetsov. Holomorphic extension of the logistic sequence. Moscow University Physics Bulletin, 2010, No.2, p.91-98. (Russian version: p.24-31) DOI 10.3103/S0027134910020049 | |||
</ref>. | |||
If the [[fixed point]] are com[lex (not real), the [[superfunction]] and the [[Abel function]] can be constructed with the [[Cauchi iteration]] | |||
<ref name="analuxp"> | |||
http://www.ams.org/mcom/2009-78-267/S0025-5718-09-02188-7/home.html | |||
Preprint: http://www.ils.uec.ac.jp/~dima/PAPERS/2009analuxpRepri.pdf | |||
D.Kouznetsov. Analytic solution of F(z+1)=exp(F(z)) in complex z-plane. Mathematics of Computation, v.78 (2009), 1647-1670. | |||
</ref><ref> | |||
http://tori.ils.uec.ac.jp/PAPERS/2010vladie.pdf | |||
D.Kouznetsov. Superexponential as special function. Vladikavkaz Mathematical Journal, 2010, v.12, issue 2, p.31-45. | |||
</ref><ref name="uniabel"> | |||
http://www.springerlink.com/content/u7327836m2850246/ | |||
http://tori.ils.uec.ac.jp/PAPERS/2011uniabel.pdf | |||
H.Trappmann, D.Kouznetsov. Uniqueness of Analytic Abel Functions in Absence of a Real Fixed Point. Aequationes Mathematicae, v.81, p.65-76 (2011) | |||
</ref>. With superfunction <math>F</math> and the Abel function <math>G</math>, the non–integer (and even complex) iterate of the transfer function <math>T</math> is straightforward; any holomorphic function can be declared as a [[transfer function]] and iterated any number of times by formula (6). | |||
In 2011, [[Henryk Trappmann]] had suggested an example of a [[transfer function]] that has no [[fixed point]]s, let it be called [[Trappmann function]] or tra; <math>\mathrm{tra}(z)=z\!+\!\mathrm e^z</math>. Such a transfer function was supposed to be difficult to iterate. However, the [[superfunction]] | |||
( | (say, "SuperTrappmann") can be expressed through that for function [[zex]], <math>\mathrm{zex}(z)\!=\!z\exp(z)</math>; then the "SuperTrappmann" can be called simply [[hen]], and the iterates | ||
<math>\mathrm{zex}(z)\!=\!z\exp(z)</math> | of the [[Trappmann function]] can be expressed with (6) at <math>F\!=\!</math>[[hen]] and <math>~G\!=\!\mathrm{ahe}=\mathrm{hen}^{-1}</math>. The iterates of [[zex]] are shown in Figure 3, and the iterates of the [[Trappmann function]] tra are shown in Figure 4. | ||
It seems, that the non-integer iterates can be defined for any holomorphic function, but the rigorous mathematical proof of such conjecture is required. | It seems, that the non-integer iterates can be defined for any holomorphic function, but the rigorous mathematical proof of such conjecture is required. | ||
In some cases, the non–integer iterate can be defined also | In some cases, the non–integer iterate can be defined also through the [[Schroeder function]]. | ||
In general, the non-integer iterate is not unique, and the specific behavior of the superfunction <math>F</math> should be assumed for the | In general, the non-integer iterate is not unique, and the specific behavior of the superfunction <math>F</math> should be assumed for the uniqueness. | ||
==Examples== | ==Examples== | ||
| Line 106: | Line 147: | ||
===Elementary functions=== | ===Elementary functions=== | ||
Let | Let | ||
| Line 121: | Line 160: | ||
More "solvable" | More "solvable" transfer functions can be constructed using [[Table of superfunctions]]. | ||
However, for some cases the solution cannot be simply expressed with elementary functions. Few of them are mentioned below. | However, for some cases the solution cannot be simply expressed with elementary functions. Few of them are mentioned below. | ||
| Line 162: | Line 201: | ||
==References== | ==References== | ||
{{reflist}} | |||
==Keywords== | ==Keywords== | ||
[[Superfunction]], | [[Superfunction]], | ||
[[Abel function]], | [[Abel function]], | ||
[[Transfer equation]], | [[Transfer equation]], | ||
[[Abel equation]] | [[Abel equation]] | ||
Latest revision as of 07:27, 9 September 2020
The iterate of a function , or iterate (итерация), is a function, expressed as repetition of another (iterated) function; the latter may be called the iterand.
Any function by itself is considered as its first iterate.
The zeroth iterate of a function is considered to be the identity function.
The minus first iteration of a function is its inverse function
In general, iteration of a function is denoted with superscript; means the th iteration of function , i.e.,
(1)
where the function in the right hand side of equation is repeated times. (Note, however, that this abstract notation is not always used consistently for actual functions: for example, cos2θ means (cos θ)2, not cos cos θ, by convention.)
This may be expressed with the equations
(2) , for
Term iteration may have also another meaning, indicating repetition of some process (even if such a repetition does not define a new function).
Physical sense
If is transfer function of some device, say, amplifier, then, the transfer function of combination of two such devices can be expressed as ; and the transfer function of combination of identical devices can be written as [1].
Nest
In Mathematica, the special operation is incorporated, called Nest. Usually, Nest has 3 arguments. The first argument is name of iterand, which is function. The second argument is initial value of the iterations, and the last argument is number of iteration. With Nest, the iteration of function can be written as follows:
(3)
In Mathematica, all arguments of all functions should be written in squared parenthesis. Up to year 2012, the Nest is implemented only for the cases, when number of iterations can be simplified to a natural number, expressed with integer constant. Attempts to call Nest to evaluate a non–integer iterate of a function are interpreted as error.
Non–integer iterate
The non–integer iterate is simple and obvious in the case when the iterand is linear function; then the iteration can be expressed through the elementary function. Let . Then
(4)
This expression allows the straightforward interpretation for non–integer .
The case of power function is also simple. Let , where . Then . In this expression, has no need to be integer. For , the iterations of quadratic function are shown in Figure 1.
For more general case, the non–integer iterates of a function can be defined through its superfunction and the Abel function ; the iterand is treated as the transfer function, and the superfunction satisfies the transfer equation
(5)
Then, the th iteration of the transfer function can be expressed as
(6)
In this expression, parameter also has no need to be integer. Once holomorphic functions and are established, the function can be iterated even complex number of times. For a given transfer function , the establishment of the superfunction and the Abel function is not trivial, but solvable problem. It had been formulated in 1950 by Helmuth Knezer [2] for iterates of exponential, the half-iterate of the exponential appears in the title of his article. In century 21, such a function had been calculated and plotted [3][4]; the iterates of the exponential are shown in Figure 2. Then, the formalism had been extended to other functions, in particular, to the exponentials to other bases [5][6], factorial [1]and the logistic sequence [7].
For the non–integer iterates, it should be assumed that is holomorphic function, and the superfunction is also expected to be holomorphic. In order to provide uniqueness of the superfunction, the certain requirements on its behavior should be assumed. Usually, approaches some fixed points of function at infinity (see the special articles Superfunction and Abel function).
Usually, the physically–meaningful transfer functions have a real fixed point and the corresponding superfunction and the Abel function can be evaluated with the regular iteration [5][6], factorial [1]and the logistic sequence [7]. If the fixed point are com[lex (not real), the superfunction and the Abel function can be constructed with the Cauchi iteration [3][8][9]. With superfunction and the Abel function , the non–integer (and even complex) iterate of the transfer function is straightforward; any holomorphic function can be declared as a transfer function and iterated any number of times by formula (6).
In 2011, Henryk Trappmann had suggested an example of a transfer function that has no fixed points, let it be called Trappmann function or tra; . Such a transfer function was supposed to be difficult to iterate. However, the superfunction (say, "SuperTrappmann") can be expressed through that for function zex, ; then the "SuperTrappmann" can be called simply hen, and the iterates of the Trappmann function can be expressed with (6) at hen and . The iterates of zex are shown in Figure 3, and the iterates of the Trappmann function tra are shown in Figure 4.
It seems, that the non-integer iterates can be defined for any holomorphic function, but the rigorous mathematical proof of such conjecture is required.
In some cases, the non–integer iterate can be defined also through the Schroeder function.
In general, the non-integer iterate is not unique, and the specific behavior of the superfunction should be assumed for the uniqueness.
Examples
This section offers a little bit more detailed description of figures, mentioned above.
Elementary functions
Let
The corresponding superfunction and Abel function are
,
give the iterate
More "solvable" transfer functions can be constructed using Table of superfunctions.
However, for some cases the solution cannot be simply expressed with elementary functions. Few of them are mentioned below.
Special functions
Figure 1 shows iterates of quadratic function, . In this case, the th the iterate can be expressed through the power function.
Figure 2 shows iterates of the exponential, . In this case, for non–integer , the th iterate
is expressed through tetration tet and ArcTetration ate, and cannot be easy represented through elementary functions.
Figure 3 shows iterates of function zex, . In this case, for non–integer , the th iterate
can be expressed through functions SuZex and AuZex, which are, of course, superfunction and Abel function for the transfer function zex.
Figure 4 shows iterates of the Trappmann function, . In this case, for non–integer , the th iterate
is expressed through the Henryk function hen and the ArcHenryk function ahe , and cannot be easy represented through elementary functions. However functions hen and ahe can be expressed in terms of SuZex and AuZex mentioned above in the following way:
and
The number of examples can be greatly extended, using the Table of superfunctions, that offers triads , and general expression .
In this way, one can plot the iterates of exponential to various bases, iterates of factorial, iterates of the logistic operator, and iterates of any functions, assuming, that we can choose the appropriate superfunction and the Abel function. In principle, any holomorphic function can be iterated any number of times, even complex number of times.
This article is adopted from TORI [10].
References
- ↑ 1.0 1.1 1.2 http://www.springerlink.com/content/qt31671237421111/fulltext.pdf?page=1 http://tori.ils.uec.ac.jp/PAPERS/2010superfae.pdf D.Kouznetsov, H.Trappmann. Superfunctions and square root of factorial. Moscow University Physics Bulletin, 2010, v.65, No.1, p.6-12. (Russian version: p.8-14)
- ↑ http://tori.ils.uec.ac.jp/PAPERS/Relle.pdf H.Kneser. "Reelle analytische Loesungen der Gleichung f(f(x))=ex und verwandter Funktionalgleichungen". Journal fur die Reine und Angewandte Mathematik 187 (1950): 56-67.
- ↑ 3.0 3.1 http://www.ams.org/mcom/2009-78-267/S0025-5718-09-02188-7/home.html Preprint: http://www.ils.uec.ac.jp/~dima/PAPERS/2009analuxpRepri.pdf D.Kouznetsov. Analytic solution of F(z+1)=exp(F(z)) in complex z-plane. Mathematics of Computation, v.78 (2009), 1647-1670.
- ↑ http://tori.ils.uec.ac.jp/PAPERS/2010vladie.pdf D.Kouznetsov. Superexponential as special function. Vladikavkaz Mathematical Journal, 2010, v.12, issue 2, p.31-45.
- ↑ 5.0 5.1 http://www.ams.org/journals/mcom/2010-79-271/S0025-5718-10-02342-2/home.html http://tori.ils.uec.ac.jp/PAPERS/2010sqrt2.pdf http://tori.ils.uec.ac.jp/PAPERS/2010q2.pdf D.Kouznetsov, H.Trappmann. Portrait of the four regular super-exponentials to base sqrt(2). Mathematics of Computation, 2010, v.79, p.1727-1756.
- ↑ 6.0 6.1 http://www.ams.org/journals/mcom/0000-000-00/S0025-5718-2012-02590-7/S0025-5718-2012-02590-7.pdf http://tori.ils.uec.ac.jp/PAPERS/2012e1eMcom2590.pdf H.Trappmann, D.Kouznetsov. Computation of the Two Regular Super-Exponentials to base exp(1/e). (Mathematics of Computation, 2012 February 8.) ISSN 1088-6842(e) ISSN 0025-5718(p)
- ↑ 7.0 7.1 http://tori.ils.uec.ac.jp/PAPERS/2010logistie.pdf http://www.springerlink.com/content/u712vtp4122544x4 D.Kouznetsov. Holomorphic extension of the logistic sequence. Moscow University Physics Bulletin, 2010, No.2, p.91-98. (Russian version: p.24-31) DOI 10.3103/S0027134910020049
- ↑ http://tori.ils.uec.ac.jp/PAPERS/2010vladie.pdf D.Kouznetsov. Superexponential as special function. Vladikavkaz Mathematical Journal, 2010, v.12, issue 2, p.31-45.
- ↑ http://www.springerlink.com/content/u7327836m2850246/ http://tori.ils.uec.ac.jp/PAPERS/2011uniabel.pdf H.Trappmann, D.Kouznetsov. Uniqueness of Analytic Abel Functions in Absence of a Real Fixed Point. Aequationes Mathematicae, v.81, p.65-76 (2011)
- ↑ http://tori.ils.uec.ac.jp/TORI/index.php/Iteration
Keywords
Superfunction, Abel function, Transfer equation, Abel equation

















![{\displaystyle ~~~f^{n}(z)=\mathrm {Nest} [f,z,n]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/8a6c12f7b1f4ce31ddbec9276f2c53c179560baa)






























